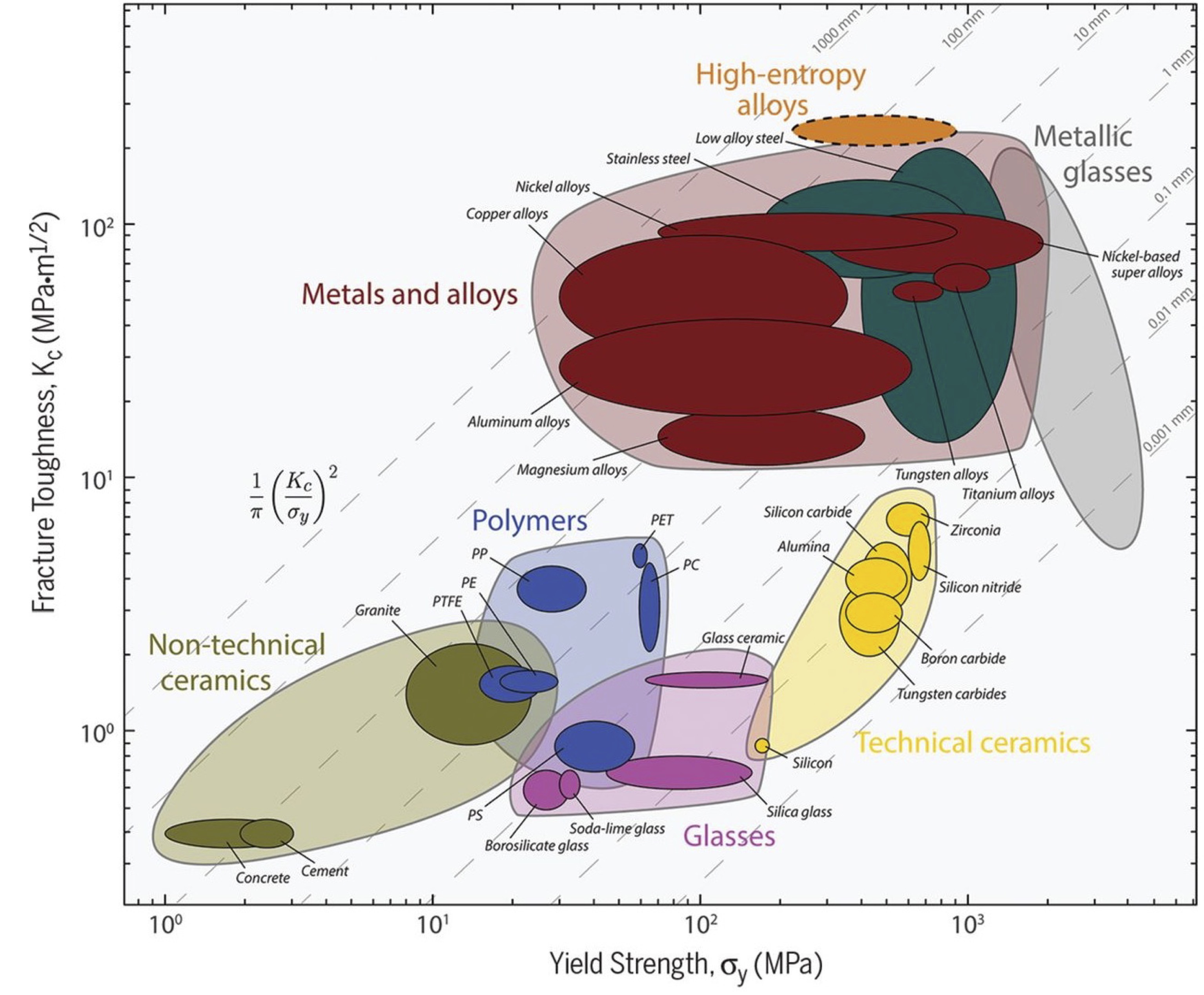
Since their inception in 2004, high entropy alloys (HEA) have attracted a great deal of attention due to a unique combination of properties seldom found in other material types. This makes them potentially very attractive for a number of technological applications. As an example, the figure below shows an Ashby diagram of the fracture toughness vs the yield strength, showing generic HEA as superior to almost all other materials [1]. The basic idea for creating these alloys is to combine a number of elements (typically five or more) in similar proportions and achieve solid solution phase stability through the large configurational entropy of the system. Due to the large chemical and configurational space available to create these materials, several hundred different HEA combinations now exist, each with their own distinct compositions, structure, and properties. The large volume of research on the topic over the last decade has resulted in a fast-evolving field full of new findings, unexplained results, and also of unresolved controversies. Note that, while the term "multicomponent" or "multielement" alloys is sometimes preferred in the literature over "high entropy" alloys (particularly when the number of elemental constituents is less than five), here we use all of them interchangeably.

Among the different systems proposed, refractory high entropy alloys (RHEA) are a special class of alloys composed of typically four or more refractory metal elements (Nb, Mo, Ta, V, W, Cr, Hf, Zr). While compositionally complex, these systems generally crystallize into a simple body-centered cubic (bcc) phase, found to be stable up to very high temperatures. Similar to their pure bcc metal counterparts, RHEA may suffer from a lack of ductility in the low temperature regime. However, they retain very high strengths at high temperatures, making them attractive candidates for high temperature structural applications, such as in the aerospace and nuclear industries. By way of example, the next figure shows compression test results for the Nb25Mo25Ta25W25 system [2], clearly illustrating both of the points just discussed. The alloys are seen to exhibit a remarkable degree of ductility above 800oC, coupled to yield strengths in excess of 500 MPa. At room temperature, however, the system displays poor ductility and high strength.

While the temperature dependence of the strength of bcc metals and their alloys is relatively well understood, no theory exists that explains the behavior observed in body-centered cubic RHEA. Figure 3a shows the dependence of the yield stress with temperature for several classes of refractory multielement alloys. An interesting feature of these single-phase systems is the observation of a temperature-insensitive region at intermediate temperatures, preceded by a more or less sharp drop from the value at 0 K, and followed by a gradual decline to retained strengths of several hundred MPa. This is in contrast with the observed temperature dependence of the flow stress in pure bcc metals, which follows a monotonic decrease from its value at a few Kelvin (300 to 1000 MPa, depending on the material) to a few MPa at the athermal limit (approximately a quarter of the melting point). Bcc metal plasticity at low to medium homologous temperatures is known to be controlled by the thermally-activated glide of screw dislocations on close-packed planes by way of kink-pair nucleation and propagation. In single crystals, the response can often be reduced to the behavior of a single dislocation source, which has resulted in a direct connection between atomistic calculations of single screw dislocation properties and the macroscopic response. It has also been shown that at high temperatures and/or strain rates screw dislocations undergo self-pinning due to the formation of cross-kinks, i.e., topological conflicts arising from collisions of kinks residing on different glide planes. Extensions to dilute bcc solid solutions exist, although to a much smaller measure and only for a few selected systems. In all, our understanding of the fundamental slip behavior of dislocations and their effect on bcc metal plasticity is quite robust at present, and its main principles can be used to guide research in other bcc alloys. However, multicomponent random alloys introduce a new paradigm due to their high compositional complexity and varied atomic chemical interactions. In such environments, even the equilibrium structures of screw dislocations are not clearly understood (their dynamic behavior even less so). Standard theories of solid solution hardening are insufficient to describe the fine details of local compositional fluctuations, which are thought to govern dislocation properties in these systems. For example, cross-kinks may be formed at stresses and/or temperatures much lower than in pure metals or dilute alloys, assisted by the existence of a large density of lattice mismatch points along the dislocation line, which arrests kinks and enhances kink collisions. This could result in considerable self-pinning, which may help explain the behavior of certain RHEA such as Nb25Mo25Ta25W25, which, as shown in the next figure [3], displays a temperature-insensitive yield strength in the 600 to 1200oC interval, and retain strengths of over 400 MPa at 1600oC.
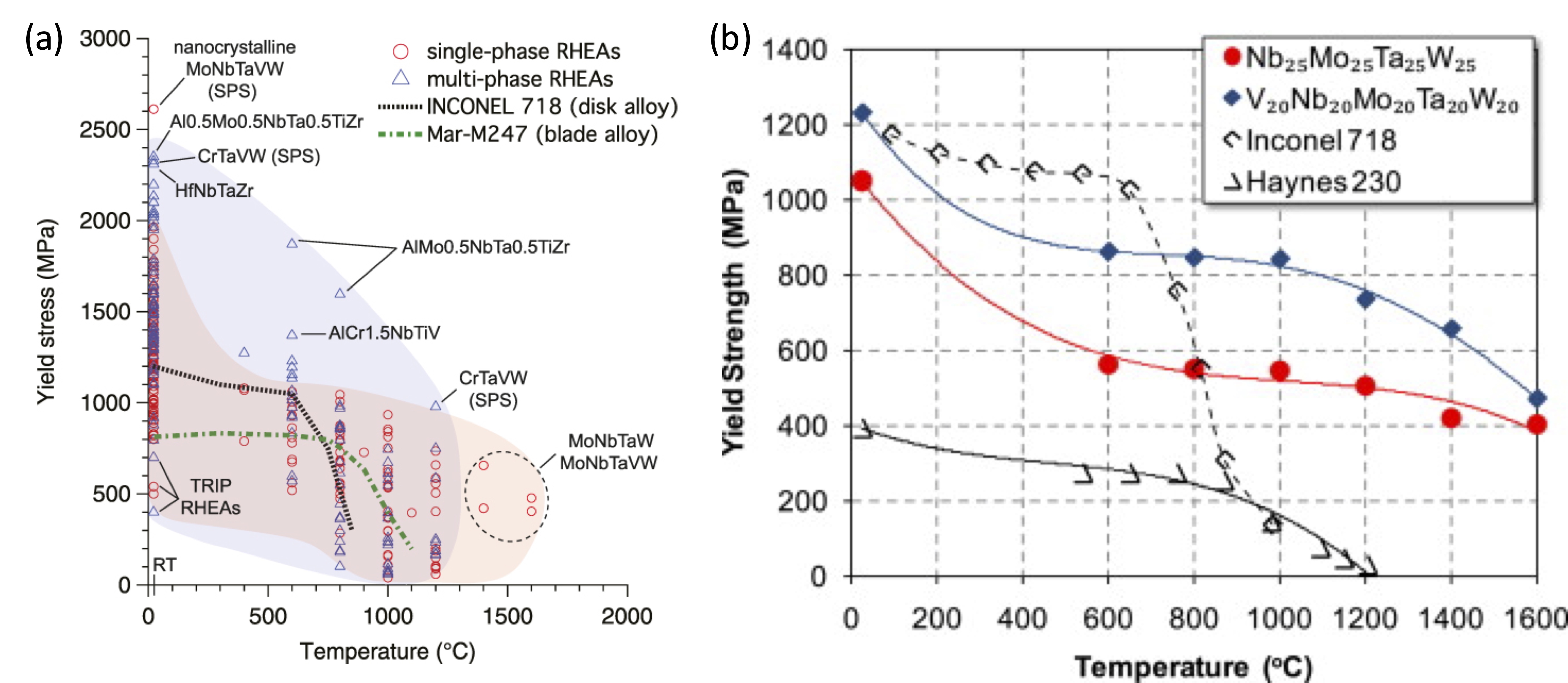
Additionally, size-scaling studies can be a useful tool to investigate unit mechanisms in small-scale pillars, 'starving' the material of volumetric dislocation sources and using the pillar diameter to set the length of the mobile dislocation sources. This also avoids the effect of back stresses caused by dislocation patterning, as has been observed recently for several RHEA. These experiments also provide a simple way to study single-crystal deformation to allow the investigation of the crystallographic orientation dependence. However, while these studies provide an ideal validation testbed for the models due to the simplicity in geometry and loading conditions, only a handful of such studies exist for Nb-Mo-Ta-W alloys, most of them focused on columnar thin films and nanocrystalline specimens. Similarly, no measurements of strain rate sensitivity --usually a reliable predictor of dynamic strain ageing-- exist for single crystals of these alloys.
In the Marian Group we are working on several fronts to try to gain insight into the mechanisms that contribute to the high-temperature strength in these alloys. On the simulation front, we are working on both the properties of screw and edge dislocations, and their dynamics in RHEA as it relates to non-conservative motion as a way to partially explain their high temperature proiperties. For this, we use a combination of kinetic Monte Carlo models, Discrete Dislocation Dynamics, and Moelcular Dynamics simulations to understand the relevant mechanisms contributing to the alloy's strength. For this, sometimes we use model alloys with a reduced complexity, such as the 'medium' entropy system Nb-Ta-V. On the experimental side, we perform a number of different analysis to understand how chemical compositional fluctuiations correlate with (macroscopic) alloy strength by using nanocompression tests within a STEM, and coupled to EDS (electron dispersive spectroscopy). Also, we carry out high-resolution TEM in order to image dislocations and extyract their character, glide planes, and Burgers vectors.
1. B. Gludovatz, A. Hohenwarter, D. Catoor, E. H. Chang, E. P. George, R. O. Ritchie, "A fracture-resistant high-entropy alloy for cryogenic applications", Science 345 (2014) 1153-1158.
2. O. N. Senkov, G. B. Wilks, J. M. Scott, D. B. Miracle, "Mechanical properties of Nb25Mo25Ta25W25 and V20Nb20Mo20Ta20W20 refractory high entropy alloys", Intermetallics 19 (2011) 698-706
3. Y. Zhang, "Microstructures and properties of high entropy alloys", Progress in Materials Science 61 (2014) 1-93.